October 5, 2016 k-2-operations-and-algebraic-thinking
Connecting Ten Frame Activities to Number Bonds and Number Sentences, K–2
By: Jeff Todd
When I visit classrooms, I often see students engaged in wonderful fun activities, but I can’t help noticing that these activities don’t always connect to the math ideas that the teacher is hoping to explain. I have worked hard in my own teaching to help students make the connection between fun and understanding, and I hope this post gives you some insight into how I do this.
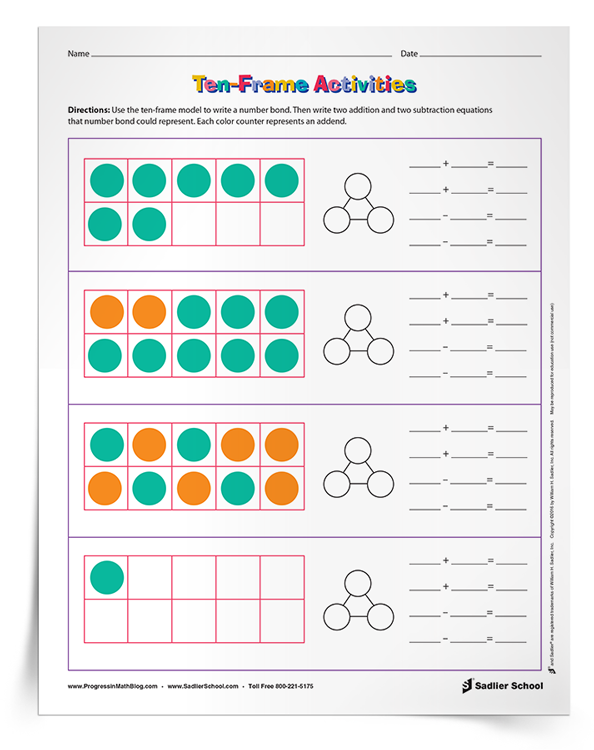
I will be using ten frame activities as an example of how manipulatives can be used to develop an understanding of addition and subtraction number sentences. The download for this activity includes two ten frame activities where students write number bonds and number sentences that go with their ten frame.
I hope you’ll bear with me as I give a bit of the history of what I have learned about teaching students using manipulatives. In the 1960s, Jerome Bruner coined the terms enactive, iconic, and symbolic to describe how students progress from using manipulatives, to making drawings based on the manipulatives, to using numbers and symbols alone. Today, we might call these steps concrete, representational (semi-concrete), and abstract. Singapore Math uses the terms concrete, pictorial, and abstract. These three sets of terms all refer to the same core strategy of using manipulative with mastery to show a mathematical idea, then having students represent that idea using paper and pencil (re-presenting it), and, finally, using only numbers and symbols to represent it.
Using ten frame activities is popular with teachers in the early elementary math classroom. Students use the counters as manipulatives with the ten frames. Assuming that students have mastered ten frame activities, you can then use the frames to start writing number bonds. The first thing I suggest that you do to help students move to the next level of development in math is to write a number bond that corresponds to the ten frame. Number bonds are a semi-concrete (pictorial) representation of the ten frame. For instance, you could have the following situation in a ten frame:
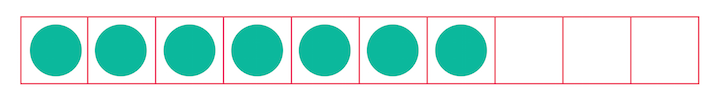
Depending on how you have been teaching number bonds, you might have displayed two colors of chips in the ten frame, seven red chips and three blue ones. I chose to represent seven blue chips in the frame and three blanks.
Students can re-present the mathematical idea in the ten frame as a number bond. This would be the semi-abstract or pictorial representational of the ten frame and is the second of the steps in the process outlined above.
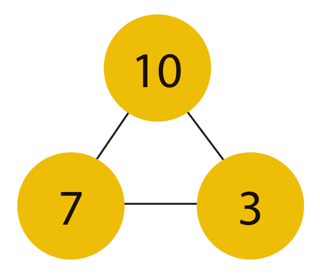
Once students have mastered the creation of number bonds from the ten frame activities, they are ready to move to more abstract representations. Ask students, “How would you write a number sentence based on the number bond?” and, “Can you write a different number sentence from that number bond?” Cue students by asking them to use addition or subtraction sentences if they can’t come up with number sentences for both operations. The four number sentences related to the number bond above are:
7 + 3 = 10 3 + 7 = 10 10 - 3 = 7 10 - 7 = 3
This is just one way for you to help students move from the use of counters to number sentences. It’s important to be patient and give students time to forge these connections, as they can be difficult to learn and shouldn’t be rushed. I hope you can see how using ten frames and manipulatives can be connected to writing number sentences, using number bonds as an intermediate step.
The download includes two ten frame activities—the first is a page with several completed ten frames where students can write the number bond and the related number sentences. The second page is a blank template that students can use to create their own problems.